Oh, manipulatives. We have all heard of them and we all have an opinion on them. Whether they are sitting on our shelves or stuffed in a closet…it’s time to talk about math manipulatives. What makes them so important? And why do we need to start using them?

If you were to poll teachers about why math manipulatives are important, you will probably get a lot of answers that lie in the realm of child/brain development. Many will say it’s about the kinesthetic, hands-on experience. And while this is true, it’s only one side of the coin when it comes to the importance of math manipulatives. Kinesthetic hands-on experiences can help students be more engaged in lessons, therefore increasing their attention and retention of knowledge. But this is true for any content…what makes manipulatives important in MATH? How do they help students understand math?

Screen Shot 2021-07-10 at 9.01.41 PM.png

A word you will always hear in the conversation around manipulatives is concrete. But what does that mean? There is a misconception that concrete means tangible. In math, a natural way to explain quantitative phenomena, everything is abstract. I like to explain concrete as what is left when you strip everything unnecessary away and just get down to the MATH. Like if you tore a house down to the concrete foundation, this is what concrete math is. The MATH. CONCRETE does not necessarily mean hands-on.

It is more about how you use manipulatives to construct visual understanding and help students explain their thinking.

Digital manipulatives and even drawings are just as good, mathematically - they only lack the kinesthetic experience and FUN of hands-on manipulatives.

When you provide hands-on tools (manipulatives) for students, you get the best of both worlds: a kinesthetic, fun experience that helps build quality visuals to understand a concept. THIS is why manipulatives are so vital. But you must be careful that you aren’t turning manipulatives into a rote step-by-step activity. Then you are missing the math side of the coin and only reaping the benefits of the kinesthetic, hands-on fun.

Take this quote from Julie Sarama and Douglas H. Clements in their article, Physical and Virtual Manipulatives: What Is “Concrete”?

“It is not just symbols that are too often learned by rote. Students often learn to use manipulatives by rote. They perform the correct steps but have learned little more about the manipulation of quantities. One student used bean and bean sticks to model place value, but used the one bean as a ten and the sticks as a one. In a similar study, kindergarten students could not use cubes to help them solve simple addition and subraction problems. They did not have a strategy to use the cubes to solve the problems. Using a number line was even more difficult. These and other studies prove an important point. Although they provide support and mediation, manipulatives do not carry mathematical ideas directly to the leaner. Although kinesthetic experience can enhance perception and thinking, understanding does not travel through the fingertips and up the arm.”

Julie Sarama and Douglas H. Clements

So, if we need to help students build conceptual understanding through visuals…and manipulatives can help - what manipulatives do we need?

Unfortunately, the answer also isn’t concrete.

The best manipulative for a student for a certain concept is….the manipulative that works best for that student for that concept. Whatever material will help them create a quality visual representation, build understanding, and explain.

Have a variety of manipulatives and don’t limit their potential. Visuals are the important aspect here.

But this doesn’t necessarily mean you need a TON of manipulatives, even if it may seem like it. I hoard manipulatives like they’re going out of style but that is because I am me and also because I like to have a variety of options for students who like different things (use stuff kids like - engagement) or identify concepts with different manipulatives than their peers.

But because the manipulative itself doesn’t really matter….you can have 1-2 and still get a great result. Again, it’s not the manipulative doing the work…it’s the BRAIN.

Screen Shot 2021-07-10 at 9.09.00 PM.png

BUT…I will tell you my favorites.

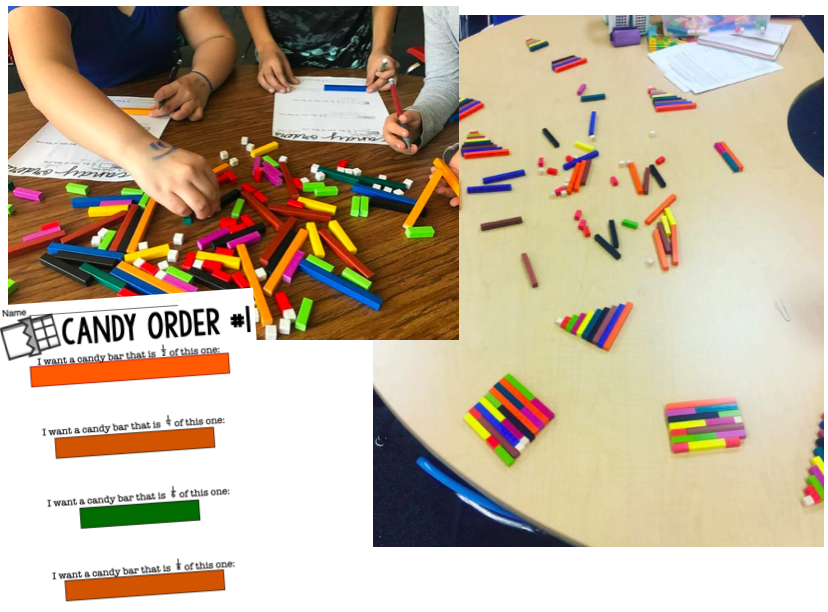
1) Cuisenaire rods are AMAZING because they are basically quantity without the number element. We let numbers take the driver’s seat way too much in math, to the detriment of algebraic thinking. These rods help students think algebraically. They can be used to build concept of quantity, balance equations, think about the concept of more/less/equal, addition, multiplication, fractions, etc. Learn more about this wonderful manipulative in my blog post on algebraic thinking in elementary here!

Screen Shot 2021-07-10 at 9.13.10 PM.png

2) Unifix Cubes (or anything that kids can snap together and take apart) are necessary for unitizing - an important skill for understanding our base-ten number system and for multiplicative thinking. This manipulative can also be used as counters, base ten blocks, etc. Connect them into groups for multiplication, connect them together to measure and compare objects, etc. The possibilities are endless.

Screen Shot 2021-07-10 at 9.16.46 PM.png

3) Counters are a favorite because….they can be anything. The classic red and yellow, the fun translucent ones, teddy bears, buttons, or Target erasers. Just something for students to work with QUANTITY and again, strip back the numbers. This is important when encountering new concepts, like multiplication (make groups instead of just writing numbers and symbols!)

4) Inch Tiles are the one manipulative I sent home with students for virtual learning last year. This is a manipulative that can give you the most bang for your back. They can be used as counters, they can make tens (in sticks OR frames), use them to build area and perimeter, measure units of inches, etc. This manipulative is another multi-use gem!