Oh hey, here we are again, talking about my favorite topic: making kids THINK. Let’s get into it.

At the heart of thinking tasks lie the mathematical process skills, including reasoning, problem solving, communicating, conjecturing, and representing. These skills empower students to think critically, analyze information, communicate their ideas effectively, and explore the intricacies of mathematical concepts. Moreover, they go hand in hand with general good thinking skills, such as collaborating, listening actively, sharing ideas, and reflecting on one's own thought processes.

One effective strategy for designing thinking tasks is to take existing activities and transform them into opportunities for deep thinking. By flipping traditional activities, we can infuse them with elements of open-ended exploration, encouraging students to delve into rich problem-solving experiences.

Let's consider a traditional numeracy task where students practice multiplication using flashcards or worksheets. To design a thinking task, we can flip it by presenting students with a numerical pattern and asking them to identify the rule or equation that generates the pattern. This task encourages students to think critically, make observations, and identify mathematical relationships. It goes beyond simple memorization of multiplication facts and invites students to actively engage in problem-solving and pattern recognition. By providing students with opportunities to uncover patterns and think flexibly, we foster their mathematical reasoning skills and promote a deeper understanding of multiplication concepts.

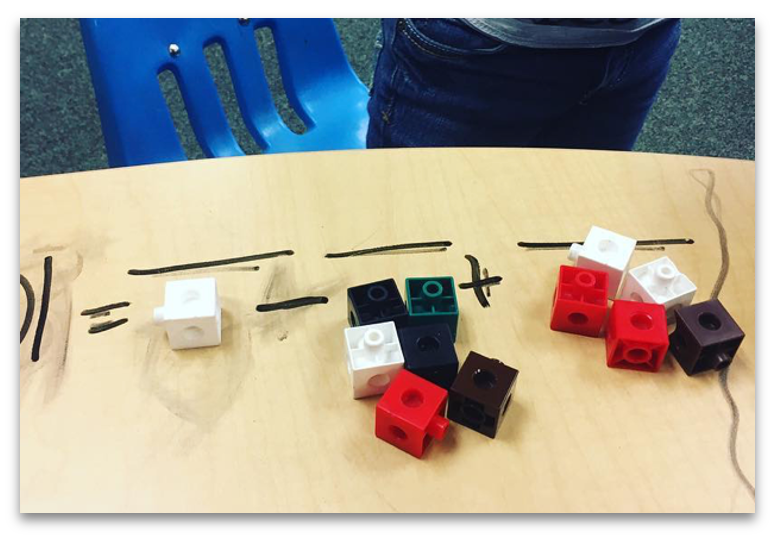
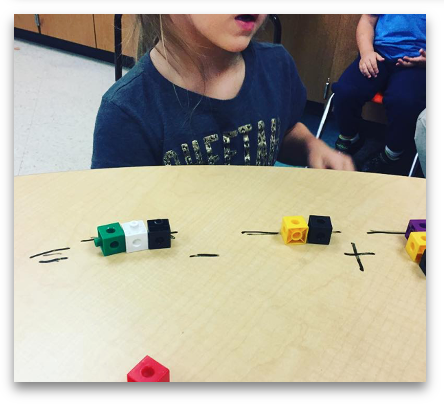
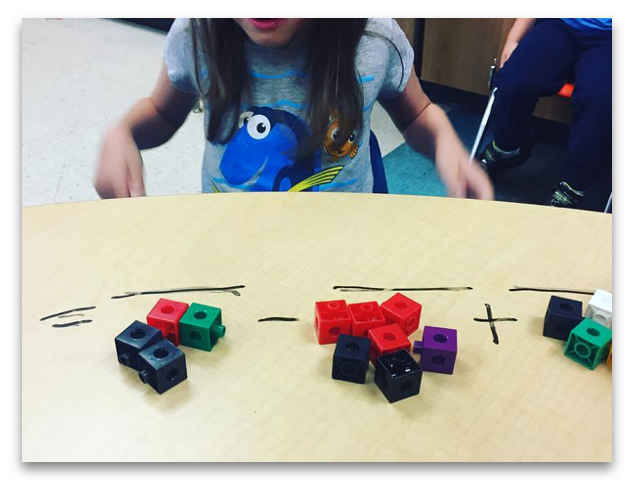
My first year of teaching was with kindergartners. During small group time, I decided to try out a new activity with them. I drew an "open equation" on the table and had them use blocks to make the equation true. Some students had ___ + ___ = 6, others had ___ + ___ + ___ = 10, or even ___ + ___ - ___ = 10. To my surprise, this activity quickly became their favorite during small group sessions. It not only engaged them but also showcased their remarkable ability to think deeply about mathematical concepts. It was a powerful reminder that even our youngest students are capable of high cognitive thinking and can thrive when given the opportunity to engage in meaningful tasks.

When first introducing thinking tasks, it is essential to start with tasks that are slightly lower in complexity and not directly tied to specific content or math standards. This approach allows students to experience success, build confidence, and develop their thinking skills without feeling overwhelmed. By emphasizing the importance of the thinking process rather than the final answer, we create a supportive environment that nurtures students' intellectual growth and fosters a love for learning.

Here are some examples of good thinking tasks that aren't necessarily math-content related:

Provide students with a list of unrelated words or objects (e.g., banana, shoe, cloud, clock). Challenge them to come up with as many unique and imaginative ways these items could be connected or combined.
Present students with logic puzzles or tasks that require them to deduce information based on given clues. For example:
- Mary is older than Jane. Jane is older than Sarah. Who is the youngest among them?
- There are five houses in a row, each painted a different color. The red house is to the left of the blue house. The green house is next to the yellow house. Which color house is in the middle?
Offer students puzzles that involve visual patterns, rotations, or transformations. For example, ask students to determine the missing piece in a sequence of shapes or to identify the next shape in a pattern.

These tasks are designed to facilitate that mindset shift for students. They allow time for students to start working on those thinking skills as well as collaboration.

After students have started shifting their mindset and are comfortable with the routine, you can incorporate tasks related to your current concept. Take a look at your curriculum or unit plans and ask yourself, "What is the concept here?" and "What is the math students need to be doing?" Then you can look for or create tasks that address the mathematical skills alongside sense-making.

Let's say you are working on a unit on area and perimeter. Traditionally, you might want students to solve the area and perimeter of many different shapes. You may even let them work in groups or play a game. But how could we still have them working on the concepts of area and perimeter and using deep thinking skills?

Consider providing tasks like these:

You have a length of fencing material that is 30 feet long. Your task is to create as many different rectangular enclosures as possible using the entire length of the fencing material.
Imagine you are designing the floor plan for your dream house or an amusement park. Create a floor plan that includes different rooms or attractions. Calculate the area of each room or attraction and consider how you can optimize the use of space. Be creative and make sure to label your dimensions.
Crystal decides that she wants fringe put all the way around the rug. If the rug maker puts fringe around the rugs, how much fringe will he need for each rug? Use pictures, equations, or words to model your thinking.
Jana is designing a daycare center for small dogs. She wants to design different rectangular pens for her dogs to play. Each pen must have a total area between 49 and 100 square feet.
What is the biggest perimeter you can make with a rectangle that has an area of 24?

You can see that some of these tasks are perfect for the beginning of the unit, and some are great for after students have been taught some vocabulary. The beauty is that the point of these tasks isn't just the mathematical thinking but the process skills they practice.

Similarly, when teaching a unit on place value, you could have students work on problems where they fill in the hundreds, tens, and ones of certain numbers. But what if you had them try these tasks:

Dylan has 634 cubes in a pile. How many groups of a hundred could he make? Any left over? How many groups of ten could he make? Any left over?
Pencils come in cases of 100, packs of 10, or as single pencils. Show me what an order of 283 would look like.

Designing tasks doesn't always have to be laborious or difficult. It can be as simple as flipping your task from asking for a solution to asking for a question. For example, in a unit on rounding, instead of asking what 83 rounds to, try asking students to make a list of numbers that are close to 83 and far from 83. Be sure to have them defend and explain their thinking.

By incorporating these different types of thinking tasks into your teaching, you can create a dynamic and engaging learning environment that promotes critical thinking, problem-solving, and mathematical reasoning. These tasks not only help students develop their thinking skills but also foster a love for learning and a deeper understanding of mathematical concepts. So, embrace the power of thinking tasks and unlock the potential of your students' minds!

Happy math-ing!